From 21 to 23 August, the Workshop on Geometrical Aspects of Material Modelling will be held in the Sala de Grados of the Faculty of Education of the Universidad Nacional de Educación a Distancia (UNED). ‘It is an interdisciplinary meeting that combines differential geometry, the mechanics of deformable solids and the physics of materials to study how to describe and predict the behaviour of materials based on their geometric structure,’ say the organisers, including Manuel de León, research professor at the Spanish National Research Council (CSIC) at ICMAT.

Ágata Timón García-Longoria (ICMAT)

When a rubber is stretched, it elongates, but when the force is released, it returns to its original shape. This is an example of an elastic response, which is part of the mechanical response of the material. Another example is the plastic response – which is observed when force is applied to a piece of clay: it deforms and does not return to its original shape when the force is removed. Mathematical modelling makes it possible to characterise, in geometrical terms, the properties of a material in its mechanical response.

‘This is the case with liquid crystals – ubiquitous in everyday life – whose model is a differentiable variety with a given direction that is included in its constitutive law, or ceramic materials – used in aircraft nozzles. Biological tissues can also be analysed in this way, as cellular tissues. With these tools we can study muscles and arteries, tumour growth or processes of growth and ageing of materials,’ explains Manuel de León, research professor at the Spanish National Research Council (CSIC) at ICMAT and co-organiser of the Workshop on Geometrical Aspects of Material Modelling, together with Marcelo Epstein (University of Calgary, Canada) and Víctor Manuel Jiménez (Universidad Nacional de Educación a Distancia, UNED).

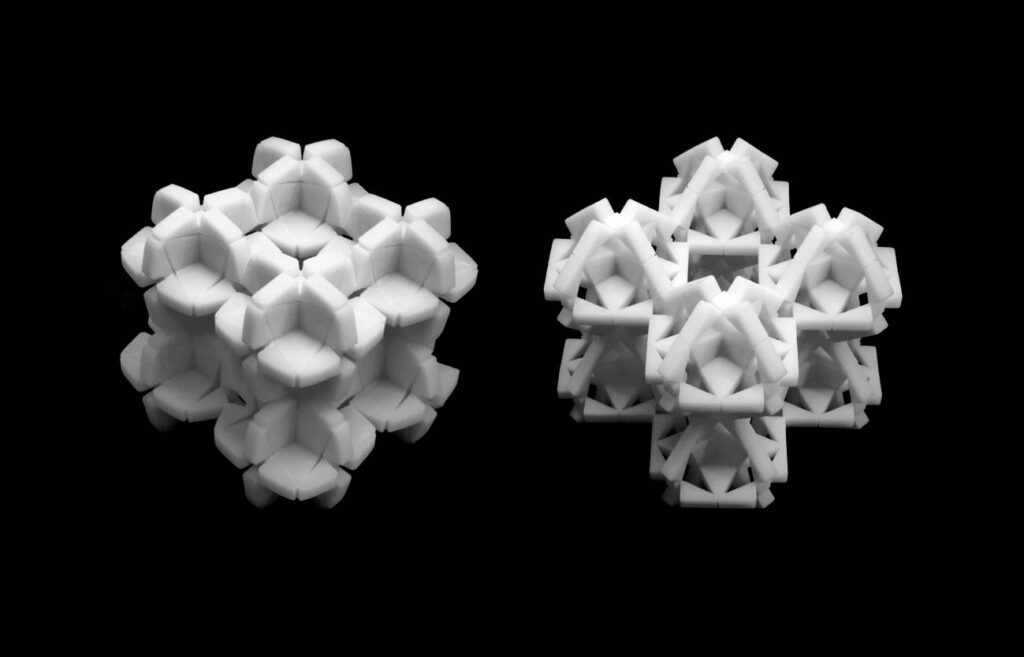
Metamaterials are designed to have specific properties, such as achieving a morphological change when loads are applied and maintaining the desired shape when the load is removed. Image: UC3M

The meeting, which takes place from 21-23 August in the UNED Faculty of Education’s Sala de Grados, will share recent advances in the field and encourage possible applications in a wide range of areas, from composite materials to biological tissues. Participants include Paolo Maria Mariano (University of Florence, Italy) – who has applied the study of neuronal plasticity to Parkinson’s disease -, Marcelo Epstein (University of Calgary, Canada) – who has made fundamental theoretical contributions, with applications in different fields of biophysics -, or Alfio Grillo (University of Torino, Italy) – author of applications to the mechanics of growth and remodelling of biological tissues -.

All of them use advanced mathematical tools -such as differential varieties, Lie groupids and differential topology- to develop models that capture the geometric complexity of materials -capable of dealing with a variety of phenomena, ranging from elasticity and plasticity to fracture and creep of materials-.

‘There are two fundamental properties in continuum mechanics that can be measured geometrically, using differential geometry (G-structures and more modernly, grupoids): uniformity and homogeneity,’ explains De León. ‘Uniformity says whether a body is made of the same material at all points: if you can transplant a small piece of material from one point to another without the mechanical response of the material changing, there is uniformity. That transplantation, in mathematical terms, is a linear transformation between tangent spaces, and the material response is given by the constitutive law (basically, the potential energy). Homogeneity is the absence of defects,’ he adds.

One of the main advantages of this approach is its ability to capture and represent the microstructure of materials at different scales, from the atomic to the macroscopic scale. This allows understanding how the geometrical organisation of atoms, grains and phases affects the macroscopic properties of the material.

With this perspective, mathematical characterisations can be used to design materials with specific properties. ‘Nowadays, metamaterials are very popular, and you can basically design and build them to have the properties you want,’ says De León.

This content has been automatically translated. The original text may differ slightly.